Ethnomathematics
Expert reviewers
Essentials
- The Western concept of mathematics with which we are all so familiar is by no means the only one
- Many, if not all, ancient cultures developed some sort of mathematical system
- It is important to appreciate and understand the worth and merit of these systems from ancient but often living and evolving cultures as occur in indigenous communities today.
- Ethnomathematics can be more relevant in some communities and cultures than ‘Western’ mathematics
- An understanding of different ethnomathematic systems can enhance our greater understanding of mathematics, so we are not limited by western perspectives alone.
All cultures are underpinned by some sort of science—some way of classifying and explaining the world and its phenomena. These systems can be based in more practical contexts, with direct applicability to everyday life, while others are more abstract and academic. In the Westernised world, we have all grown up with a certain idea of what ‘mathematics’ is, without paying all that much attention to the various complex and intricate mathematics systems that evolved with other cultures.
The term ethnomathematics was used in the late 1960s by a Brazilian mathematician, Ubiratan D'Ambrosio, to describe the mathematical practices of identifiable cultural groups. Some see it as the study of mathematics in different cultures, others as a way of making mathematics more relevant to different cultural or ethnic groups, yet others as a way of understanding the differences between cultures. But perhaps the most powerful claim for the newly recognised discipline has been made by D’Ambrosio himself:
Mathematics is absolutely integrated with Western civilization, which conquered and dominated the entire world. The only possibility of building up a planetary civilization depends on restoring the dignity of the losers and, together, winners and losers, moving into the new. [Ethnomathematics, then, is] a step towards peace.Ubiratan D'Ambrosio, The Chronicle of Higher Education, 6 October 2000
This makes ethnomathematics a rather unusual discipline, because it attempts to meld science and social justice. This is not something that sits comfortably with many scientists: science, they argue, is science, and trying to make it politically correct will only impede its progress. Some educators fret that teaching mathematics using an ethnomathematical approach reduces it to a social-studies subject that teaches students little about ‘real’ mathematics. Others simply ridicule the whole notion: according to one disparaging journalist, ‘Unless you wish to balance your checkbook the ancient Navajo way, it's probably safe to ignore the whole thing’.
However there are also many scientists, educators and commentators who see ethnomathematics—in all its definitions—as a legitimate discipline with plenty to offer the modern world.
Number system converter
Take a look at a few of the world's ancient number systems, and see how different they are from each other.
The number in is:
Convert another number
Learn more about these number systems from the Wichita State University website. Note that the systems persisted over long periods, so they evolved over time and can often be seen written in different ways. Hieroglyph/symbol image source: Wikipedia.
Examples of non-Western mathematics
Many non-Western cultures have developed complex mathematical systems. One often-cited example is that of the ancient Mayans. This civilisation, which emerged more than 3,000 years ago, recognised many patterns in their observations of the universe, and developed mathematical relationships and symbolic systems to describe these patterns. The Mayans were keen astronomers who developed a complex system of calendars to keep track of the solar and lunar cycle and other planetary events.
The counting system used to support the calendars was based on cycles of 5 (quinary) and 20 (vigesimal). (The number system predominantly used today is decimal, based on composite units of ten.) Some historians suggest that the vigesimal system derived from the total number of digits on a person’s hands and feet (just as the decimal system probably arose from finger-counting). The Mayan representation of its counting system is elegant in its simplicity. It uses only three symbols: dots, which represent single units; lines, which represent units of five; and a shell-like symbol, representing a place that could hold a number. The three symbols could be combined to represent any number.
When the Spanish conquered central America in the 1500s they destroyed many artefacts of the Mayan civilisation, including religious icons and texts. One of the few surviving texts is called the Dresden Codex—after the city in which it now resides. This document reveals the sophistication of the Mayan's knowledge of mathematics and astronomy.
Despite invasion, the Mayans remain a strong cultural group today. Importantly, many of the mathematical systems developed by their ancestors—such as counting and calendars—are still being used.

Counting systems in Papua New Guinea
Counting systems based on composite units of 5 and 20 are also common in Papua New Guinea. The 800 different language groups have their own counting systems with a variety of basic number words. Commonly used number words are hand as 5, and person (10 fingers and 10 toes) as 20. A few groups have a hand as 4 (without the thumb) or as 6 (with the thumb as two knuckles).
The counting systems in Papua New Guinea are best described in terms of the cycles (rather than the base) that they use. For example, if pairs were important to a language group, then the counting system might feature a 2-cycle, with six objects being thought of as three groups of two. Many systems would probably have a second cycle and many counting words were combinations of the frame number words (e.g 1, 2 and 5—much as we use the 10 numbers 0 to 9 in higher numbers). The second cycles are commonly cycles of five so that, for example, the number 14 might be two fives and two twos. Many systems have cycles of 2, 5 and 20.
Several language groups use parts of their bodies—up one arm, across the head and down the other arm—to mark off different numbers. These counting systems are known as body tally systems.
However, there are not always clear links to physical or cultural aspects in the Papua New Guinean counting systems. Many groups use words that are not related to parts of the body, and combinations of counting words may not be linked to specific cultural activities.
There are a variety of similar systems across Indigenous Australia.
Indigenous Australians
Mathematics is used by different cultures for activities in addition to counting. For example, the complex interpretation of natural data using (among other things) some fundamental mathematics has ensured the survival of the indigenous Australians people in the challenging Australian environment for at least 50,000 years.
Some examples of the traditional mathematics of Australian Aboriginal and Torres Strait Island peoples include counting in non-decimal systems, recognition of the patterns in relationships between clans, and calendars based on natural changes in the environment. For example, a 'season' may be defined by the sets of natural phenomena that occur at a given time, such as the flowering of particular plants, the activities of bees and the direction of the wind.
Other mathematics of the indigenous Australians is also based on the relationships between things. A spear may be ‘too long’ for a particular person, too short or just right: the length of the spear is thus measured relative to the user.
The island navigators
The Polynesians, Micronesians and Melanesians, who populated thousands of islands scattered across the western, central and southern Pacific Ocean, needed a different kind of mathematics. Without a reliable navigation system, sailing and paddling between islands perhaps thousands of kilometres apart would be highly dangerous. The Marshall Islanders, for example, used a combination of techniques when they ventured onto the high seas, complementing celestial navigation—using the moon, the sun and the rising and setting of specific stars—with a detailed understanding of wave and current patterns.
Learning to read wave patterns in the ocean swell was not an easy skill to master. The Marshallese knew that the swell, which was created by the reliable trade winds and could run in a straight line for great distances, was reflected, refracted and diffracted when it met islands, setting up patterns in the waves that could be ‘read’ to help determine position.
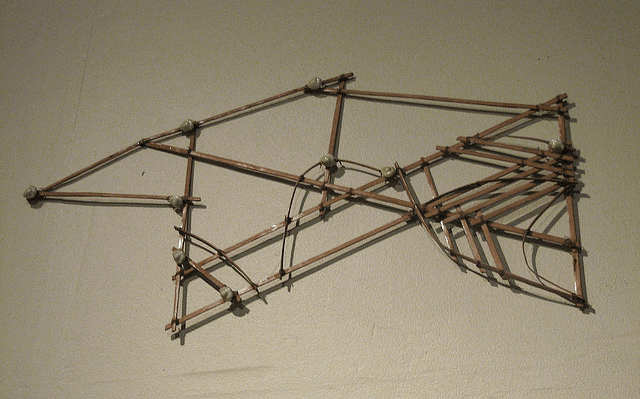
Over the centuries, the Marshallese acquired a great deal of knowledge about wave patterns and how they were affected by islands and island groups and by changes in wind direction, but without a written language it was difficult to pass such knowledge to young navigators. They solved this problem with stick charts, lashing sticks together to form geometric shapes illustrating the patterns of currents and waves that would be encountered on a given voyage. There were different charts for different purposes and also stone charts for star charts. The stick charts were used as a visual aid for budding navigators, who would spend years learning these and other patterns, forming the mental maps of the ocean that they would need as they made their way from one tiny atoll to another.
Visuospatial reasoning
The study of ethnomathematics has also shown that many non-western cultures use strong visuospatial reasoning to influence their decision making.
- Visual
- picturing images and concepts in the mind, but may also be external such as viewing a diagram or plan
- Spatial
- thinking about space, position, movement, orientation, and also using bodily movements to recognise spatial situations.
- Reasoning
- may be instantaneous, practiced or learned, encompassing recognition, use of pattern and movement to assist in decision making, and making mental comparisons with past examples and experiences.
Essentially, visuospatial reasoning is the ability to visualise spatial concepts and images in the mind (though may also be external such as viewing a diagram or plan), and then manipulate and reason with these images based on past experience.
For many Indigenous people around the world, these visual images are directly related to the everyday lived experience of their culture. For example, a parallelogram is a shape obtained by tying two longer sticks and two shorter sticks end to end and then skewing them to the right or left. Parallelograms do not have a name in Papua New Guinea culture, however these shapes tend to tell people that a house is going to fall, or it is used in weaving because it is aesthetically pleasing. Another example involves the use and application of practical trigonometry through the use of knots and ropes to build bridges to cross rivers.
Dr. Kay Owens notes that ‘visuospatial reasoning is apparent in designs distinct to different PNG cultures including continuous lines, symmetries, similarities, and angle sizes occurring in carvings, string bags, painted faces and bodies and woven walls.’ Visuospatial reasoning can also relate to equivalents, ratios, determining angles and associated lengths associated with, for example, the slopes of roofs; areas of grass or plant size etc. Similarly, length of string or part of the forearm can be linked to the volume of a bilum (string bag), or the girth of a pig to its volume and hence its mass. While visual reasoning dominates over numerical reasoning, numbers will still be used on occasion.
While these are not the traditional mathematical ‘absolutes’ that the Western system is used to working with, they are a valid way of looking at problems, and solving them. The integration of these different systems can enhance learning and even change perceptions of what mathematics is, while strengthening its relevance to everyday life.
What's the point?
Some people maintain that ancient mathematics systems are irrelevant today. This is unfortunate. Many non-Western mathematics systems remain ‘alive’; some Mayans, for example, still use traditional calendars for religious purposes and to help determine the agricultural cycle, while Indigenous groups in Papua New Guinea and Australia continue to use ethnomathematic concepts in their everyday life.
Moreover, Western mathematics does not meet the needs of all people and is not always easily understood outside the ‘mainstream’ culture. For years, Australian educators have noted that Western mathematics often has little meaning in remote Aboriginal communities and is therefore difficult to communicate. Approaches that take into account the cultural context and the mathematical systems in use within the community are likely to be much more effective, as students’ cultural identity can be harnessed to encourage their mathematical identities. The Aboriginal and Torres Strait Islander Mathematics Alliance (ATSIMA) noted that ‘schools need to recognise mathematics in culture, celebrate and validate culture and recognise culture as mathematical’.
Incorportating ethnomathematics into the school curriculum can not only result in better grades for students, it can facilitiate powerful mathematical processes such as visualising mathematical situations, encouraging a sense of proportional size, recognising mathematics in practice, making links between home and school, and encouraging relevant, daily problem solving.

Conclusion
There is great value, and much to be learned from the ethnomathematic principles of Indigenous cultures. By allowing ourselves to see beyond the Western numbering system, we can try to increase our understanding of not only ancient cultures, but also to see the world, and many of the mathematical problems in it, in a different, yet equally as relevant way.





